Selling through lotteries: an art to combat ambiguity

There's a chill in the air, but the sun is out. Your best friend has just messaged you: "This is the perfect weather for getting out and into the countryside." You walk past your local bike shop and notice there's a mountain bike on sale. You think to yourself: "Wow, that's way below the price I was expecting." You go in and confirm the price with the shop assistant. You think to yourself: "A bargain." — You buy the bike!

You've had enough of the fresh air. You go back online. You see a popup message on your screen. There's an opportunity to pay for opening treasure chests with a high chance of buying rare in-game items. The price is at an attractive discount. You think to yourself: "A bargain." - You click on it!

From the seller's perspective, what are the motivations behind these different selling mechanisms? Selling a product to a customer whose valuation of the product is the customer's private information is a fundamental problem in revenue management. The key decision herein is to decide an optimal pricing scheme that maximises the seller's expected revenue, given the practice that customer valuation, often unobservable, is typically modelled as a random outcome from certain probability distribution.

The posted price mechanism

Announcing a fixed price to the customer, formally termed the posted price mechanism, is a simple yet powerful way to sell the product. In the posted price mechanism, it is assumed that for any given price, a customer whose valuation is above the price would buy the product. That is to say, the optimal posted price would maximise the seller's expected revenue by maximising the product of (1) a deterministic price and (2) the probability that the customer valuation is not smaller than that price. The practice of the posted price mechanism commonly appears, e.g., in brick-and-mortar stores. Indeed, it is a celebrated result in mechanism design that whenever the probability distribution of customer valuation is known to the seller, the posted price mechanism is optimal among all possible selling mechanisms the seller shall consider.

Alternative ways of selling

Of course, there are many other ways to sell. For example, the seller can sell a lottery: the customer pays a fee to enter the lottery and wins the product with certain probability. Alternatively, the seller can randomise the price (e.g., by rolling the dice): the seller posts a distribution of prices and randomly draws one price from the distribution. An interesting fact is that although framed in perspectives that seem to be totally different, selling using lotteries and randomised pricing are mathematically equivalent! It is also worth noting that the posted price mechanism is a special case of the selling through lotteries mechanism, where the customer pays an entrance fee that is high enough would win the product for sure! At first glance, selling using lotteries (and/or randomised pricing) is less intuitive than the posted price mechanism. Nevertheless, the practice of using lotteries as a selling mechanism has been commonly seen in the online game industry, for instance, in the form of drop rates of rare in-game items.

Is there any incentive for a seller to use lotteries?

Let us consider the following simple example. The customer values the seller's product at $2 or $1 or no value at all. We assume here that the customer would purchase the product if she is indifferent between purchasing and no purchasing; and the customer would choose to pay the posted price if she is indifferent between buying the product and buying the lottery. The seller is aware of the possible values and is also confident that the average valuation is at $1. However, the seller does not have any further information on the probability at each possible value: $0, $1 or $2.

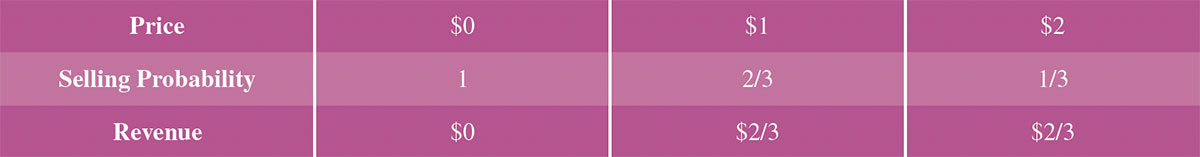
Suppose the seller presumes a uniform distribution over the values $0, $1 and $2. Then the optimal selling mechanism (under the hypothesised distribution) is to post a price of $1 or $2 with the hypothesised optimal revenue of $2/3; see Table 1.

However, if in the true distribution the customer values the product at $0 or $2 each with a probability of one half (with the mean valuation stays at $1), then with the posted price of $1 that is optimal under the hypothesised distribution, the seller's expected revenue would drop to $1/2 because the customer would buy the product with a half chance.

Consider now the following selling through lotteries mechanism. The seller posts a price of $4/3 and simultaneously sells a lottery priced at $2/3 with a winning probability of 2/3. Clearly, a customer with valuation zero would not purchase anything. A customer who values the product at $1 would prefer to buy the lottery because her valuation $1 is smaller than the posted price $4/3; while under the selling through lotteries mechanism, her net utility, i.e., the difference between her valuation times the winning probability and the price of lottery, amounts to $1×2/3 - $2/3 and is not smaller than $0. Using similar calculations, we can tell that the customer with valuation of $2 would pay the posted price of $4/3. One can then show that under the selling through lotteries mechanism, the seller's revenue is guaranteed to be $2/3, as long as the customer's valuation is distributed over the values of $0, $1 and $2 with an average valuation of $1.

In the above example, the power of the posted price mechanism is limited when facing the ambiguity in the probability distribution of the customer's valuation. Here, the ambiguity refers to the fact that the precise probability distribution is not known to the seller. This phenomenon is often the case in practice. In many realworld applications, the probability distribution of the customer's valuation is rarely known precisely, but rather, the seller only has a confident prediction of, at best, certain statistics of the customer's valuation, such as the maximum the customer is willing to pay or the average valuation of population. In such a case, the posted price mechanism may be suboptimal.

Is there a better mechanism that the seller can use?

The answer is a firm "YES!" The example above shows that the seller can indeed do better and achieve a robust revenue guarantee of $2/3 if he uses the selling through lotteries mechanism beyond the posted price mechanism. This motivates us to study the general problem of finding a robust selling mechanism when the seller has limited information on the valuation distribution.

Instead of having a perfect knowledge on the probability distribution of the customer's valuation, we assume the seller merely knows that it belongs to a family (termed the ambiguity set) of probability distributions that share certain identical statistics that are deemed reasonable and are easy to estimate through historical sales data or surveys. The seller then solves a max-min problem that seeks to maximise the worst-case revenue over all possible probability distributions in the ambiguity set.

Building upon the famous minimax theorem in game theory, for a very general ambiguity set, we show that the problem of finding the optimal selling through lotteries mechanism is equivalent to a min-max problem in which an adversary seeks to find a worstcase distribution to minimise the maximum revenue achievable by a posted price mechanism. Although in the min-max problem, the seller, who originally considers the selling through lotteries mechanism, is now restricted only to a posted price mechanism, he enjoys the information advantage of first seeing the distribution chosen by the adversary before choosing his own price. Our result demonstrates that the extra value brought by more sophisticated selling mechanisms over the simple posted price mechanism is exactly the value of information under a posted price mechanism. Quite interestingly, depending on the ambiguity set, the extra value can be just zero or can approach to infinity!

Our findings that show the selling through lotteries mechanism can outperform the posted price mechanism reveal the potential of revenue improvement from randomisation, when facing ambiguity in the probability distribution of the customer valuation. From this perspective, we once again confirm one of the most important takeaways in the field of decision-making under uncertainty: randomisation based on a carefully designed device, as an art to combat ambiguity, may lead to significant improvement over deterministic decisions. So, when launching your new product next time, please do remember to bring the dice with you!